Ball on a rotating turntable Hugh Hunt http://www.eng.cam.ac.uk/~hemh
|
|
|
|
|
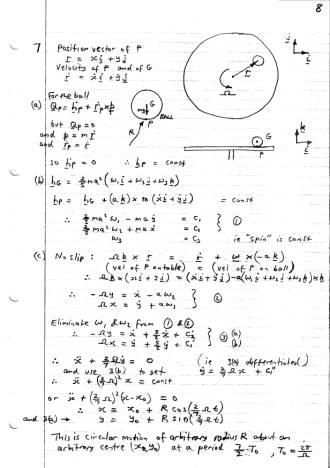
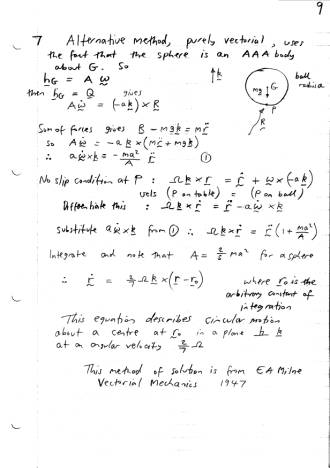
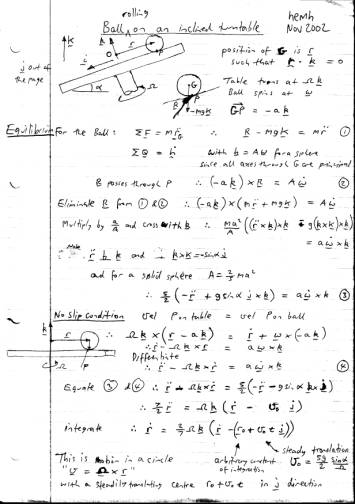
The dynamics of a ball on a rotating table have been understood for decades. An elegant and compact vectorial treatment can be found in Milne, E. A.”VECTORIAL MECHANICS”. The analyses given here (top right and bottom left) are based on this vectorial approach. The analysis given
above (top left) for a ball on a horizontal rotating table is purely
Cartesian and is easier for those less skilled in vector manipulation to
follow. It makes use of |