Moment of momentum measures an objects tendency to continue to spin, it describes the rotary inertia of a system in motion about an axis.
The moment of momentum, h0 about a fixed point o is defined as
Where
r is the position expressed as a displacement vector from the origin
x represents the vector cross product
mv is the linear momentum (mass x velocity)
Conservation of moment of momentum
If there is no resultant applied moment or torque about an axis then moment of momentum will be conserved about this axis. This is because Newton's Second Law for rotational motion about a fixed axis can be expressed as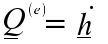
where h is the
moment of momentum. Therefore, if Q=0
(i.e. there is no applied moment
or torque) then 
= 0
and so moment of momentum is conserved.
Conservation of moment of momentum applied to gyroscopic motion
For a precessing gyroscope so long as there is no resultant applied moment about the vertical axis (frictionless conditions) moment of momentum is conserved about the vertical axis (marked on the diagram below).
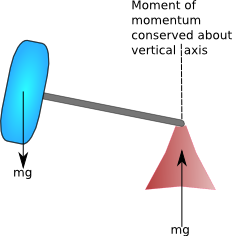
However, if friction is present, as is often the case in practice this can provide a resultant applied torque about the vertical axis and so moment of momentum will not be conserved. Conservation of moment of momentum may be a valid assumption if this friction is very small, but this will not always be the case.