onetofour

Demonstration of gyroscopic precession

In the early part of his lecture Laithwaite uses a Victorian gyroscope to demonstrate gyroscopic precession. Precession describes a change in the direction of the axis of a rotating object, so in this case a change in the spin axis of the gyroscope.

Laithwaite hangs weights from a Victorian gyroscope in order to demonstrate precession

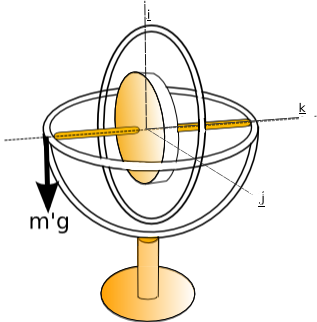
Diagram of Victorian gyroscope used by Laithwaite to demonstrate precession

The weights that Laithwaite hangs of the gyroscope are represented by m'g where m' is their total mass.

When the weight is added to the gyroscope (with the rotor spinning) gyroscopic precession occurs. This precession is in a direction perpendicular to the direction that the weight force acts. The precession that occurs is demonstrated in the animation on the left which shows a view from above the gyroscope as it precesses (parts of the gyroscope such as the base are omitted for clarity)

Why does precession occur?

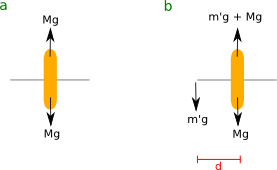
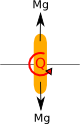
The weight that is hung of the gyroscope (represented by a cross on the diagram to the left) is offset from the center of mass of the gyroscope and stand. It is this offset of forces which causes precession.

Side view of gyroscope showing offset of forces

It can be seen that prior to hanging the weight off the gyroscope all the forces acted through the same point (the center of mass), there was no precession in this case. From b) it can be seen that all the forces no longer act through the same point and are offset by a distance, d. A couple is defined as a system of forces with a resultant moment but no resultant force. 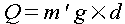Therefore b) is equivalent to a) with a moment, Q also acting, where Q=m'g x d

Side view of b) with the offset of forces represented as a moment

It can be seen that by hanging a weight of the gyroscope in the position shown a moment has been introduced into the system.

The moment of momentum of the rotor is defined as

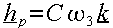where C is a constant given by the principle moment of inertia about the k axis. From this formula it can be seen that the direction of the moment of momentum vector coincides with the direction of spin of the rotor.

Using the information that the offset force can be represented as a couple and the direction of the moment of momentum vector is parallel to the spin the precessing gyroscope can also be represented as below.

When Newton's laws are applied to circular motion the following important result is obtained

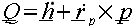
The gyroscope is mounted on a stand and so the center of the rotor is a fixed point therefore this can be simplified to

This result is key, the moment of momentum vector, h is changed a small amount in the direction of Q (the applied couple).
.

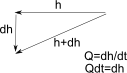
The direction of the couple and spin vectors can be calculated using the right hand screw rule. From this the direction of precession is obtained using the information that the moment of momentum and so spin axis is changed in the direction of the applied couple.

In the absence of the weight precession did not occur this was due to the fact there was no applied couple Q and so no change in the moment of momentum in other word the spin axis stayed fixed.

Precession of a toy gyroscope

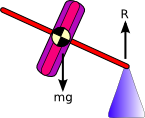
Toy gyroscopes provide a good demonstration of precession. In this case it is not an applied weight which results in a couple but the fact that center of mass of the gyroscope is not above the point of contact and so the weight force and reaction force are offset.

The offset of forces results in an applied couple, Q. The point of the gyroscope that is in contact with the top of the tower stays stationary, so here

and precession occurs about this point. The direction of precession is dependent on the direction of spin of the rotor.

Toy gyroscopes are easy to get hold of in most toy shops, so this can be tested and the direction of spin varied to see what happens to the direction of precession. The moment of momentum vector,h will always move in the direction of the applied couple.

Demonstrations of precession

Videos of precession can be seen with supporting calculations.

Nutation

Another motion nutation can also be observed during precession, thus is a steady state motion of the bodies k axis about the K axis with constant angle between them, it is observed as a fast wobbling motion

Home page

Videos

Explanation of gyroscopic precession

Newton's Laws of motion

Flash animations

Videos 1-4

Demonstration of gyroscopic precession

Why does precession occur?

Precession of a toy gyroscope

Demonstrations of precession

Nutation